- E3
-
Describe all the differentiable functions \(f:ℝ→ℝ\) that solve
\[ ∀ x~ ,~ (f'(x))^ 2 + (f(x))^ 2 = 1~ . \]Show that if \(-1{\lt}f(x){\lt}1\) for \(x∈ I\) open interval, then \(f\) is a sine arc, for \(x∈ I\).
Show that all solutions are \(C^ 1\), and that they are piecewise \(C^∞\).
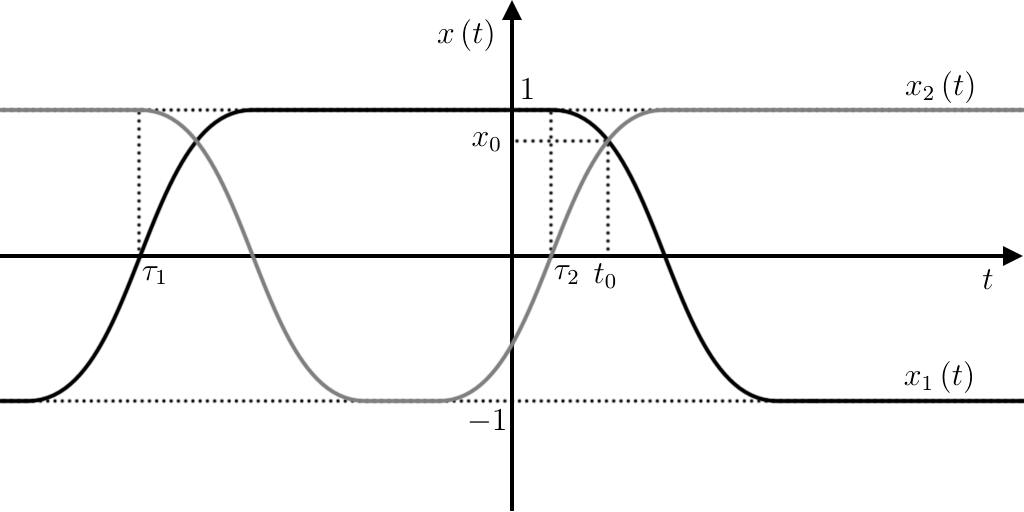
Note that \(f≡ 1\) and \(f≡ -1\) are envelopes of the other solutions, as explained in the section [1QB].
 Solution 1
Solution 1
EDB — 1QK
View
English
Authors:
"Mennucci , Andrea C. G."
.
Managing blob in: Multiple languages